PMC Module 7 Mathematical Exercises (Part 2) Solved assignment |Early Childhood (3-6) | Pakistan Montessori Council | SB Online Academy
 |
| PMC Module 7 Mathematical Exercises (Part 2) Solved assignment |
Write answers in your own words. Your
answers should be at least 2 typed pages or 3 handwritten pages. Please don’t
forget to write your name and roll number on each page of the assignment.
Q1:
Explain different groups of
Montessori Math exercises and how
the directress should efficiently present exercises through sequential and
parallel work in various groups.
Answer No 1
Montessori mathematical exercises are
normally grouped into the following
groups:
1.
Number
through Ten
2.
Decimal
System
3.
Counting
beyond Ten (Linear & Skip Counting)
4.
Memorization
of Arithmetic Tables
5.
Passage
of Abstractions
6. Fractions
There are some
sequential work and some parallel work in these arithmetic grouped exercises
which tells that how the directress should work efficiently through different groups.
·
Numbers Through Ten:
The exercises in this group are sequential.
When the child has a full understanding of numbers through Ten (numbers rods, sand paper, spindle boxes, card and
counters, golden beads bar and coloured beads bar). In this group, the child
builds the basic concept of numbers and also recognize the relationship between
quantity and numerals.
·
Decimal System:
The decimal system
can be introduced when the child has a full understanding of numbers through
ten. The focus here is on the hierarchy of the decimal system and how the
system functions. It also starts the child on the exercises of simple
arithmetic operations such as addition, multiplication, subtraction and
division. They are introduced at this
level.
·
Counting beyond Ten:
This group will be
started when the decimal system is well
underway. After that , these exercises will be given parallel to the
counting of the decimal system . In this group counting beyond the ten includes
the Teens, Tens and linear and skip counting.
The exercises in
this group can be introduced while the
later work of the Decimal System and
Linear and Skip Counting exercises are on going.
·
Passage to Abstraction:
In this group
exercises allow the child to stop
the use of Montessori material and work
on paper. As soon as the child is well familiar with tables of a mathematical
operations such as addition. He may move ahead into the abstraction phase of
that operation while learning the tables
of other operations and can begin to work more with the symbols on paper,
without using the material to find the
answer.
·
Fraction:
The sixth group
exercises can run parallel to the group
of making abstractions and the early
work with the fractions can begin with sensorial work.
Q2:
Explain the exercises which enable the child to count till 1000?
Answer No2
Linear
counting exercises help the child learn to count till 1000, along with getting familiar with
the decimal system relationships,
including the concept of squares
and cubes of numbers.
Linear counting is presented in two stages. In
the first stage the child learns to
count till 100, and in the second stage he masters counting till 1000.
This helps consolidate
children’s knowledge of counting . Up until now, they have worked with
tens and hundreds in the decimal system , However with these exercises , the y now become
familiar with the sequence of
numbers from 1 through to 1000. Counting is a monotonous
activity and tends to become mechanical
over time. Through repetition, children instinctively adopt this mechanism of counting.
Ø Presentation
1:
Material:
·
The
hundred chain (which is made up of 10
bars of ten connected to each other.)
·
The
hundred square
1. Blue
label marked 10-90
2. A red label marked 100
·
A
runner or large sized mat
Exercise: The children are invited to the chain
cabinet and shown the bars on the
shelves to discuss if they have seen
bars like these before. The directress starts by counting with them starting from the unit to the 10 bar and unrolling the runner just a
little. She knows them how to hold the
100 chain by both ends and lay it out
vertically at the bottom of the mat. The children are made to place the tray below the hundred
chain and slowly fold the chain together to create the hundred square, emphasizing that it looks
like the hundred square. The hundred
square is placed on top of the folded
100 chain to show that they are the
same. The hundred square is removed and the chain gently re-straightened.
The
directress takes out the green unit tickets and tells the children what they are called. They are
lined in a vertical line to the left of
the 10 chain. The children are then shown the blue ten tickets which are placed in a vertical line above the
unit tickets. The first ten are labeled using the unit tickets and placed to
the left of the chain.
Together
they count from 11 to 20. At the 20 mark,
the ticket that says 20 is placed to its right Counting by units, as
they continue placing the ten tickets until they reach 100. The
children are made to place the red 100
ticket to the 100. They are told , “you
have just counted to 100”.
She
asks, “How many beads are in this chain?” , they respond again with “100”. All the tickets are then counted alongside
the children : 1, 2, 3, 4, 5, 6, 7, 8, 9, 10,20, 30, 40, 50, 60, 70, 80, 90 and
100.
Then
counted backwards, 100, 90, 80, 70, 60, 50, 40, 30, 20, 10, 9, 8, 7, 6, 5, 4,
3, 2 and 1.
Ask
the child if he would like to count
again on his own, else have the child
place the material at their appropriate
places.
Ø Presentation
2:
Material:
·
A
thousand chain consisting of 100 bars of
ten
·
Ten
squares of hundred
·
The
thousand cube containers having below
tickets:
1. Green ticket
marked 1 – 9
2. Blue ticket marked 10- 90
3. Red ticket marked 100-990
4. Large green ticket marked 1000
·
A runner
Exercise:
The
directress tells children that they are going
to be looking at an even longer
chain than the 100 chain now. She then
has them unroll the runner all the way
and hold the 1000 chain. She then carries the chain to the runner, with
all of the strands laid out straight and has the children bring over the cube and the large box
on a tray over to the runner
along with the hundred squares. She tells them that they are going to try to fold the chain similar to how they did with the 100 chain. She makes a hundred and asks the child what she just make and places a hundred square
next to it, repeating until the whole chain has been folded into a hundred squares. The
children can begin to make them independently after a while.
The directress then places each of the hundred squares next to the
hundred squares she has made with the
children, before placing the hundred squares
on top of the hundred
squares made with them. She then counts
with the children to see how many hundred squares there are in total. She
continues to have the children place each hundred squares on top of each
other now similar to how she did herself earlier, and then iterates how
this now looks just like the cube, and that when we have 10 hundred
squares, we know that we have 1000 beads.
Now she places the cube next to the ten hundred square to show this to the children . She then has them gently, pull the 1000 chain straight and lay out all
of the tickets. Each bead is
counted and the correct ticket placed
as and when needed similar to presentation 1. When the number 100 is
reached, including the 1000 bead.
At the end of exercise, she looks at the
world of children , going back to the beginning and counting, 10, 20, 30, 40,
50, 60, 70, 80, 90, 100, 110, …………….., 400, 410, 420, …..980, 990, 1000.
Ask the child to count by tens backwards.
When finished have the child to return all the material.
Q3:
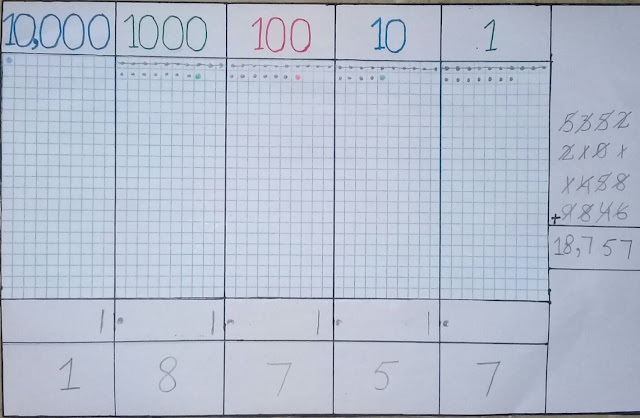
Print * Dot Game paper (from the link given below) and send three solved problems , each carrying four addends?
Answer No 3
Q4:
Explain the presentations of Multiplication Board and Division Board in your
own words. Also make illustrations.
Answer No 4
Multiplication
Board:
The purpose of multiplication bead board
is to provide training in multiplication
leading to memorization of the important multiplication table 1 x 1 through 10 x 10. The box contains a holed multiplication working, 100 holes in
rows of ten arranged in a square, 100 red beads, a box of small plastic cards
numbering 1-10 which represent the multiplicand and a red disc. The board has a
slot on the left side for card insertion.
Material:
·
Multiplication
Board.
·
A
red disc
·
A
set of cards from 1 to 10 to insert in the board
·
Printed
multiplication table sheets.
·
Multiplication
chart 1 ( summary of multiplication tables) for control of error
Exercise:
The
directress shows children the material and the numbers along the top of the board and tells them that those numbers tell us how many times to take a number. She also demonstrates how
to slide the “6” card into the slot on
the side of the board, as she tells
them, “This tells us how we will be doing the Table of 6”. She then places the
little red disc above the 1 at the top
of the board and says, “this tell us we need to take 6 one time.” Using the red
beads, she places 6 one time in a vertical line and asks the children to count the total numbers of
beads that are on the board and tells
them, “6 x 1 is 6”.
The children are then instructed to write
the answer on the paper next to the
equation. She then moves the disc over above the ‘2’ and tells the children , “we now need 6
two times, but we have already 6 one times’. The children are told to
place the red beads in a vertical line
next to the first six and hen count the total number of beads on the board. She
then says, “6 x2 is 12”. The directress repeat it till the children reach 6 x 6
and then they are told to say the equation along with the directress.
This exercise should be repeated until they have finished the board.
The directress makes the children read all
of the equation and answers are written
on piece of paper. They can check their
work on Multiplication Chart 1.
Division Board:
Division Board helps the child practice
the tables of division with the dividends 1 to 81 and the divisors 1 to 9 . The
material consist of a unit division
working board, a board containing 9 green unit skittles and 81 green plastic
beads.
Material:
·
Division
board
·
Green
Skittles (9)
·
Green
beads (81)
·
Squared
paper
·
Division
tables
·
Summary
of division tables up to 81
·
Printed
slips with division problems
·
A
glass bowl
Exercise:
The
directress shows children the material and the numbers along the top of the
board and tells them that those numbers
represent the divisors. Place a skittle
below number 1 on the board and say, “This represent that the divisor is
1”. Then take another skittle and place
below number two saying, “This means that the divisor is 2”. Repeat adding a
few more skittles. Also tell him that
the numbers on the left side will be the
answer (quotient). Pointing to the last
number also tells that the answer cannot
be larger than 9. Also tell him that the remainder at the end of division cannot be equal to or larger than the divisor. Choose a
division table i .e. 27. Ask to the children the dividend their answer should be 27. Tells
them dividend is represented by green
beads , count and place them in to the bowl. Also asks for the divisor if there is 9 then place skittles below the numbers 1 to 9 on the top
of the board.
Place the beads in the holes first fill one row and then continue placing the second row. Continue
until all the beads finish. Count the filled rows answer will be 3 . pointing
to 3 along the left side of the board says,”27 / 9 equals 3” and records the
answer.
Remove the
skittle 9 and saying ‘Let’s try with 27 / 8). Repeat the same exercise with 8 as mentioned
above. With 9 . This will give 3 rows completely filled with numbers
till 8, while the fourth row will be incomplete
with only three beads. Says, “This is the remainder”. Pointing to the beads ,
the skittles and the numbers on the left of the board, say, “27 divided by 8 equals 3
with 3 remainder. Record the answer.
Proceed with the other numbers . When you
reach 27/2 , say, “ It will not possible on the board, as on this board
we cannot have a remainder which
is bigger than the divisor”.
Q5: How is Stamp Game introduced to the child ? Also explain how subtraction problems can be solved with stamp game.
Answer
No 5
Stamp
Game:
Stamp games provides further practice in
addition, subtraction, multiplication and short division in decimal system. It
also acquaints children with long division. Following items are included in
Stamp Game Box.
Material:
·
Large
quantities of wooden squares of equal size about 1 inch square like stamp:
1.
Green
tiles marked ‘1’
2.
Blue
tiles marked ‘10’
3.
Red
tiles marked ‘100’
4.
Green
tiles marked ‘1000’
·
Green,
Red and Blue Skittles
·
Green,
Red and Blue circles
Introduction:
The directress starts by inviting the children
to come work with her and bring along
the paper, the box of wooden tiles and the tray from ‘introduction to
quantity’. She introduces the materials to the child. She shows the children
Green tile with the unit 1 written on it and tells them that this is the same
as the unit bead. Similarly she shows
them the Blue tile with the unit 10 written on it and tells them that it is similar to the ten
bar. She continues to repeat this for the tiles of 100 and 1000.
The Three Period Lesson is also carried out
with the 1, 10, 100 and 1000 tiles by showing children that when we take
out the unit 1 tiles, we place them
directly in front of the compartment where the other 1’s are. They are told
that they are going to take out 5. As they take out 5 of the unit 1 tiles, they are all placed in front of the
unit 1 compartment. They are then placed back and the children are given a few different numbers to be taken out , for
example, 4 ten, 3 hundred and 1 thousands. They are then given a larger number
and tells “Now we are going to make a
larger number. The number will have 2 units, 4 tens, 3 hundred and 1 thousand”.
As they are given each number, have them take out appropriate tiles. The
directress then counts to the check the final product and then has the children
put the tiles back into their compartments.
Subtraction:
Material:
·
The
stamp game set
·
A
pencil
· Grid paper
·
A
minus sign card
Exercise
1 ( Static Subtraction with Stamp Game): The
children are invited to come and work
with the directress who writes down on a
paper two different numbers and introduces the subtraction sign. The children
are instructed to construct the number first
and told that we are going to take the 3unit from the 4 units constructed. Have them move 3
unit off to the left side of the table , count how many units are left and
write the answer. Next, have them take 2 tens away from the 5 and move them off to the side of the table.
Count and then write how many tens are
left. Repeat for the hundreds and thousands, Finally read the answer out loud
with the children.
Exercise
2 (Dynamic Subtraction with Stamp Game):
The directress writes a first large number
and a second number under it, making sure that
the number lead to dynamic subtraction.
She says the children create the first number, as she asks
them how many units are we going to take
away. i. e. as the children stuck, she says that we are going to have to change one of the
tens for units, as she takes out ten
units and replace it with one of the tens tiles. She then has them take 3 units
away from the now 12 units and places the unneeded tiles off to the side of the
table. The directress says the child write how many units he has left. She then
repeats for the tens, hundreds and thousands, changing as when needed. Read the
answer and record it.
All this is repeated until children feel
comfortable to work alone. Children should be encouraged to pose their own problem examples and guided
in the process.








No comments:
Post a Comment